
Cubics Plane v1.0 serial key or number

Cubics Plane v1.0 serial key or number
Solution of quadratics.
With negative numbers we understand that every quadratic equation in the variable x can be written in the formwhere a, b, and c are constants. We also know that the general solution is given by the quadratic formula:

where there are two distinct real solutions if the discriminant b2 – 4ac is positive, one double real solution if the discriminant is 0, and no real solutions if the discriminant is negative.
Back in the 15th century, this was not understood. Instead, quadratic equations were classified into four different kinds depending on the signs of the coefficients a, b, and c. Since the leading coefficient a is not zero in a quadratic equation, you can always divide by it to get an equivalent quadratic equation where a equals 1, that is, x2 + bx + c = 0. This one form gives rise to four forms when you move the negative terms to the other side of the equation and when you drop zero terms from the equation:
| x2 | = | c |
| x2 + bx | = | c |
| x2 + c | = | bx |
| x2 | = | bx + c |
There are other forms, but either they have no solutions among the positive numbers or else they can be reduced to linear equations. Each of these forms required a different form of a solution. With hindsight, we see that the 15th century solutions are just special cases of the quadratic formula. One would think that the consolidation of four cases into one might be enough justification for accepting negative numbers, but apparently it wasn't. It seems to take a lot of time before people will extend their concept of number to include new entities.

Solution of cubics.
Equations of the third degree are called cubic equations. The general form of a cubic is, after dividing by the leading coefficient,As with the quadratic equation, there are several forms for the cubic when negative terms are moved to the other side of the equation and zero terms dropped.
Back in the 16th century it was a big deal to solve cubic equations. There was a great controversy in Italy between Cardano (1501–1576) and Tartaglia (1499–1557) about who should get credit for solving the cubic equation. Any book on the history of mathematics will go into the details of this fascinating controversy. What’s interesting to us, though, is that negative numbers were becoming legitimatized, a deeper insight into equations was developed, and the first inkling of a complex number appeared. Incidentally, at this time symbolic algebra had not been developed, so all the equations were written in words instead of symbols!
Cardano, in his Artiss Magnæ, or Great Art, found negative solutions to equations, and he called these numbers “fictitious”. He also noted an important fact connecting solutions of a cubic equation to its coefficients, namely, the sum of the solutions is the negation of b, the coefficient of the x2 term. At one other point, he mentions that the problem of dividing 10 into two parts so that their product is 40 would have to be 5 + √(–15) and 5 – √(–15). 
Bombelli’s investigations of complex numbers
Cardano did not go further into what later became to be called complex numbers than that observation, but a few years later Bombelli (1526–1572) gave several examples involving these new beasts. Here’s one example.
One of Cardano’s cubic formulas gives the solution to the equation x3 = cx + d as

where e = (d/2)2 – (c/3)3). Bombelli used this to solve the equation x3 = 15x + 4 to get the solution

Now, the square root of –121 is not a real number; it’s neither positive, negative, nor zero. Bombelli continued to work with this expression until he found equations that lead him to the solution 4. He determined that

and, therefore, the solution x = 4. You can check those equations are correct by cubing 2 ± √–1 to get 2 ± 11√–1.
This example is not given to show that Bombelli knew everything there is to know about complex numbers, only that he was starting to understand them.
Elliptic curve

In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point O. Every elliptic curve over a field of characteristic different from 2 and 3 can be described as a plane algebraic curve given by an equation of the form
The curve is required to be non-singular, which means that the curve has no cusps or self-intersections. (This is equivalent to the condition 
An elliptic curve is an abelian variety – that is, it has a group law defined algebraically, with respect to which it is an abelian group – and O serves as the identity element.
If y2 = P(x), where P is any polynomial of degree three in x with no repeated roots, the solution set is a nonsingular plane curve of genus one, an elliptic curve. If P has degree four and is square-free this equation again describes a plane curve of genus one; however, it has no natural choice of identity element. More generally, any algebraic curve of genus one, for example the intersection of two quadric surfaces embedded in three-dimensional projective space, is called an elliptic curve, provided that it is equipped with a marked point to act as the identity.
Using the theory of elliptic functions, it can be shown that elliptic curves defined over the complex numbers correspond to embeddings of the torus into the complex projective plane. The torus is also an abelian group, and this correspondence is also a group isomorphism.
Elliptic curves are especially important in number theory, and constitute a major area of current research; for example, they were used in Andrew Wiles's proof of Fermat's Last Theorem. They also find applications in elliptic curve cryptography (ECC) and integer factorization.
An elliptic curve is not an ellipse: see elliptic integral for the origin of the term. Topologically, a complex elliptic curve is a torus, while a complex ellipse is a sphere.
Elliptic curves over the real numbers[edit]
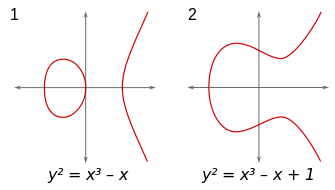
Although the formal definition of an elliptic curve requires some background in algebraic geometry, it is possible to describe some features of elliptic curves over the real numbers using only introductory algebra and geometry.
In this context, an elliptic curve is a plane curve defined by an equation of the form
where a and b are real numbers. This type of equation is called a Weierstrass equation.
The definition of elliptic curve also requires that the curve be non-singular. Geometrically, this means that the graph has no cusps, self-intersections, or isolated points. Algebraically, this holds if and only if the discriminant
is not equal to zero. (Although the factor −16 is irrelevant to whether or not the curve is non-singular, this definition of the discriminant is useful in a more advanced study of elliptic curves.)
The (real) graph of a non-singular curve has two components if its discriminant is positive, and one component if it is negative. For example, in the graphs shown in figure to the right, the discriminant in the first case is 64, and in the second case is −368.
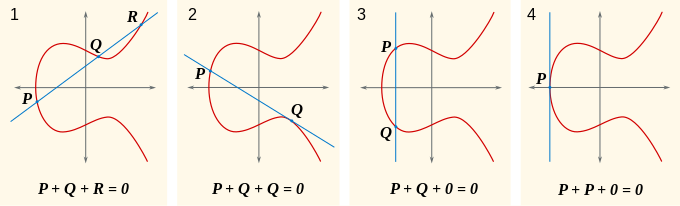
The group law[edit]
When working in the projective plane, we can define a group structure on any smooth cubic curve. In Weierstrass normal form, such a curve will have an additional point at infinity, O, at the homogeneous coordinates [0:1:0] which serves as the identity of the group.
Since the curve is symmetrical about the x-axis, given any point P, we can take −P to be the point opposite it. We take −O to be just O.
If P and Q are two points on the curve, then we can uniquely describe a third point, P + Q, in the following way. First, draw the line that intersects P and Q. This will generally intersect the cubic at a third point, R. We then take P + Q to be −R, the point opposite R.
This definition for addition works except in a few special cases related to the point at infinity and intersection multiplicity. The first is when one of the points is O. Here, we define P + O = P = O + P, making O the identity of the group. Next, if P and Q are opposites of each other, we define P + Q = O. Lastly, if P = Q we only have one point, thus we can't define the line between them. In this case, we use the tangent line to the curve at this point as our line. In most cases, the tangent will intersect a second point R and we can take its opposite. However, if P happens to be an inflection point (a point where the concavity of the curve changes), we take R to be P itself and P + P is simply the point opposite itself.
For a cubic curve not in Weierstrass normal form, we can still define a group structure by designating one of its nine inflection points as the identity O. In the projective plane, each line will intersect a cubic at three points when accounting for multiplicity. For a point P, −P is defined as the unique third point on the line passing through O and P. Then, for any P and Q, P + Q is defined as −R where R is the unique third point on the line containing P and Q.
Let K be a field over which the curve is defined (i.e., the coefficients of the defining equation or equations of the curve are in K) and denote the curve by E. Then the K-rational points of E are the points on E whose coordinates all lie in K, including the point at infinity. The set of K-rational points is denoted by E(K). It, too, forms a group, because properties of polynomial equations show that if P is in E(K), then −P is also in E(K), and if two of P, Q, and R are in E(K), then so is the third. Additionally, if K is a subfield of L, then E(K) is a subgroup of E(L).
The above group can be described algebraically as well as geometrically. Given the curve y2 = x3 + ax + b over the field K (whose characteristic we assume to be neither 2 nor 3), and points P = (xP, yP) and Q = (xQ, yQ) on the curve, assume first that xP ≠ xQ (first pane below). Let y = sx + d be the line that intersects P and Q, which has the following slope:
Since K is a field, s is well-defined. The line equation and the curve equation have an identical y in the points xP, xQ, and xR.
which is equivalent to 
We equate the coefficient for x2 and solve for xR. yR follows from the line equation. This defines R = (xR, yR) = −(P + Q) with
If xP = xQ, then there are two options: if yP = −yQ (third and fourth panes below), including the case where yP = yQ = 0 (fourth pane), then the sum is defined as 0; thus, the inverse of each point on the curve is found by reflecting it across the x-axis. If yP = yQ ≠ 0, then Q = P and R = (xR, yR) = −(P + P) = −2P = −2Q (second pane below with P shown for R) is given by
Elliptic curves over the complex numbers[edit]
The formulation of elliptic curves as the embedding of a torus in the complex projective plane follows naturally from a curious property of Weierstrass's elliptic functions. These functions and their first derivative are related by the formula
Here, g2 and g3 are constants; 

This map is a group isomorphism of the torus (considered with its natural group structure) with the chord-and-tangent group law on the cubic curve which is the image of this map. It is also an isomorphism of Riemann surfaces from the torus to the cubic curve, so topologically, an elliptic curve is a torus. If the lattice Λ is related by multiplication by a non-zero complex number c to a lattice cΛ, then the corresponding curves are isomorphic. Isomorphism classes of elliptic curves are specified by the j-invariant.
The isomorphism classes can be understood in a simpler way as well. The constants g2 and g3, called the modular invariants, are uniquely determined by the lattice, that is, by the structure of the torus. However, all real polynomials factorize completely into linear factors over the complex numbers, since the field of complex numbers is the algebraic closure of the reals. So, the elliptic curve may be written as
One finds that
and
so that the modular discriminant is
Here, λ is sometimes called the modular lambda function.
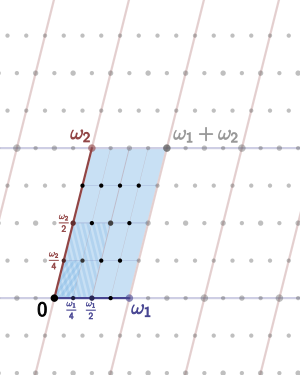
Note that the uniformization theorem implies that every compact Riemann surface of genus one can be represented as a torus.
This also allows an easy understanding of the torsion points on an elliptic curve: if the lattice Λ is spanned by the fundamental periods ω1 and ω2, then the n-torsion points are the (equivalence classes of) points of the form
for a and b integers in the range from 0 to n−1.
Over the complex numbers, every elliptic curve has nine inflection points. Every line through two of these points also passes through a third inflection point; the nine points and 12 lines formed in this way form a realization of the Hesse configuration.
Elliptic curves over the rational numbers[edit]
A curve E defined over the field of rational numbers is also defined over the field of real numbers. Therefore, the law of addition (of points with real coordinates) by the tangent and secant method can be applied to E. The explicit formulae show that the sum of two points P and Q with rational coordinates has again rational coordinates, since the line joining P and Q has rational coefficients. This way, one shows that the set of rational points of E forms a subgroup of the group of real points of E. As this group, it is an abelian group, that is, P + Q = Q + P.
The structure of rational points[edit]
The most important result is that all points can be constructed by the method of tangents and secants starting with a finite number of points. More precisely[1] the Mordell–Weil theorem states that the group E(Q) is a finitely generated (abelian) group. By the fundamental theorem of finitely generated abelian groups it is therefore a finite direct sum of copies of Z and finite cyclic groups.
The proof of that theorem[2] rests on two ingredients: first, one shows that for any integer m > 1, the quotient groupE(Q)/mE(Q) is finite (weak Mordell–Weil theorem). Second, introducing a height functionh on the rational points E(Q) defined by h(P0) = 0 and h(P) = log max(|p|, |q|) if P (unequal to the point at infinity P0) has as abscissa the rational number x = p/q (with coprimep and q). This height function h has the property that h(mP) grows roughly like the square of m. Moreover, only finitely many rational points with height smaller than any constant exist on E.
The proof of the theorem is thus a variant of the method of infinite descent[3] and relies on the repeated application of Euclidean divisions on E: let P ∈ E(Q) be a rational point on the curve, writing P as the sum 2P1 + Q1 where Q1 is a fixed representant of P in E(Q)/2E(Q), the height of P1 is about 1/4 of the one of P (more generally, replacing 2 by any m > 1, and 1/4 by 1/m2). Redoing the same with P1, that is to say P1 = 2P2 + Q2, then P2 = 2P3 + Q3, etc. finally expresses P as an integral linear combination of points Qi and of points whose height is bounded by a fixed constant chosen in advance: by the weak Mordell–Weil theorem and the second property of the height function P is thus expressed as an integral linear combination of a finite number of fixed points.
So far, the theorem is not effective since there is no known general procedure for determining the representants of E(Q)/mE(Q).
The rank of E(Q), that is the number of copies of Z in E(Q) or, equivalently, the number of independent points of infinite order, is called the rank of E. The Birch and Swinnerton-Dyer conjecture is concerned with determining the rank. One conjectures that it can be arbitrarily large, even if only examples with relatively small rank are known. The elliptic curve with biggest exactly known rank is
- y2 + xy + y = x3 − x2 − 244537673336319601463803487168961769270757573821859853707x + 961710182053183034546222979258806817743270682028964434238957830989898438151121499931
It has rank 20, found by Noam Elkies and Zev Klagsbrun in 2020.[4] Curves of rank at least 28 are known, but their rank is not exactly known.
As for the groups constituting the torsion subgroup of E(Q), the following is known:[5] the torsion subgroup of E(Q) is one of the 15 following groups (a theorem due to Barry Mazur): Z/NZ for N = 1, 2, ..., 10, or 12, or Z/2Z × Z/2NZ with N = 1, 2, 3, 4. Examples for every case are known. Moreover, elliptic curves whose Mordell–Weil groups over Q have the same torsion groups belong to a parametrized family.[6]
The Birch and Swinnerton-Dyer conjecture[edit]
The Birch and Swinnerton-Dyer conjecture (BSD) is one of the Millennium problems of the Clay Mathematics Institute. The conjecture relies on analytic and arithmetic objects defined by the elliptic curve in question.
At the analytic side, an important ingredient is a function of a complex variable, L, the Hasse–Weil zeta function of E over Q. This function is a variant of the Riemann zeta function and Dirichlet L-functions. It is defined as an Euler product, with one factor for every prime numberp.
For a curve E over Q given by a minimal equation
with integral coefficients 
The zeta function of an elliptic curve over a finite field Fp is, in some sense, a generating function assembling the information of the number of points of E with values in the finite field extensionsFpn of Fp. It is given by[7]
The interior sum of the exponential resembles the development of the logarithm and, in fact, the so-defined zeta function is a rational function:
where the 'trace of Frobenius' term[8]



There are two points to note about this quantity. First, these 





The Hasse–Weil zeta function of E over Q is then defined by collecting this information together, for all primes p. It is defined by
SpaceX Dragon
 The SpaceX Dragon approaching the ISS during the C2+ mission in May 2012. | |
| Manufacturer | SpaceX |
|---|---|
| Designer | Elon Musk |
| Country of origin | United States |
| Operator | SpaceX |
| Applications | ISSlogistics |
| Specifications | |
| Dry mass | 4,201 kilograms (9,262 lb)[1] |
| Payload capacity | to ISS 6,000 kilograms (13,000 lb), which can be all pressurized, all unpressurized or anywhere between. It can return to Earth 3,500 kilograms (7,700 lb), which can be all unpressurized disposal mass or up to 3,000 kilograms (6,600 lb) of return pressurized cargo[2] |
| Crew capacity | 0 |
| Volume | 10 cubic metres (350 cu ft) pressurized[3] 14 cubic metres (490 cu ft) unpressurized[3] 34 cubic metres (1,200 cu ft) unpressurized with extended trunk[3] |
| Dimensions | |
| Length | 6.1 metres (20 ft)[1] |
| Diameter | 3.7 metres (12 ft)[1] |
| Production | |
| Status | Retired |
| Built | 14 |
| Launched | 23 |
| Lost | 1 |
| Maiden launch | 8 December 2010; 9 years ago (2010-12-08) (first orbital flight) 22 May 2012; 8 years ago (2012-05-22) (first cargo delivery to ISS)[4] |
| Last launch | 7 March 2020 |
| Related spacecraft | |
| Derivatives | |
| Dragon RCS | |
| Fuel | NTO / MMH[5] |
The SpaceX Dragon, also known as Dragon 1 or Cargo Dragon, was a class of reusable cargo spacecraft developed by SpaceX, an American private space transportation company. Dragon was launched into orbit by the company's Falcon 9launch vehicle to resupply the International Space Station (ISS). It is now superseded by SpaceX Dragon 2.
During its maiden flight in December 2010, Dragon became the first commercially built and operated spacecraft to be recovered successfully from orbit. On 25 May 2012, a cargo variant of Dragon became the first commercial spacecraft to successfully rendezvous with and attach to the ISS.[8][9][10] SpaceX is contracted to deliver cargo to the ISS under NASA's Commercial Resupply Services program, and Dragon began regular cargo flights in October 2012.[11][12][13][14] With the Dragon spacecraft and the Orbital ATKCygnus, NASA seeks to increase its partnerships with domestic commercial aviation and aeronautics industry.[15]
On 3 June 2017, the CRS-11 capsule, largely assembled from previously flown components from the CRS-4 mission in September 2014, was launched again for the first time, with the hull, structural elements, thrusters, harnesses, propellant tanks, plumbing and many of the avionics reused, while the heat shield, batteries and components exposed to sea water upon splashdown for recovery were replaced.[16]
SpaceX developed a second version called SpaceX Dragon 2, which includes the capability to transport people. Flight testing was completed in 2019, after a delay caused by a test pad anomaly in April 2019, which resulted in the loss of a Dragon 2 capsule.[17] The first flight of astronauts on the Dragon 2, on a mission contracted to NASA, occurred in 2020.
The last flight of the first version of the Dragon spacecraft (Dragon 1) launched 7 March 2020 (UTC); it was a cargo resupply mission (CRS-20) to International Space Station (ISS). This mission was the last mission of SpaceX of the first Commercial Resupply Services (CRS-1) program. Future SpaceX commercial resupply flights to ISS under the second Commercial Resupply Services (CRS-2) program will use the SpaceX Dragon 2 version.[18]
Name[edit]
SpaceX's CEO, Elon Musk, named the spacecraft after the 1963 song "Puff, the Magic Dragon" by Peter, Paul and Mary, reportedly as a response to critics who considered his spaceflight projects impossible.[19]
History[edit]
SpaceX began developing the Dragon space capsule in late 2004, making a public announcement in 2006 with a plan of entering service in 2009.[20] Also in 2006, SpaceX won a contract to use the Dragon space capsule for commercial resupply services to the International Space Station for the American federal space agency, NASA.[21]
NASA ISS resupply contract[edit]
Commercial Orbital Transportation Services[edit]

In 2005, NASA solicited proposals for a commercial ISS resupply cargo vehicle to replace the then-soon-to-be-retired Space Shuttle, through its Commercial Orbital Transportation Services (COTS) development program. The Dragon space capsule was a part of SpaceX's proposal, submitted to NASA in March 2006. SpaceX's COTS proposal was issued as part of a team, which also included MD Robotics, the Canadian company that had built the ISS's Canadarm2.
On 18 August 2006, NASA announced that SpaceX had been chosen, along with Kistler Aerospace, to develop cargo launch services for the ISS.[21] The initial plan called for three demonstration flights of SpaceX's Dragon spacecraft to be conducted between 2008 and 2010.[22][23] SpaceX and Kistler were to receive up to US$278 million and US$207 million respectively,[23] if they met all NASA milestones, but Kistler failed to meet its obligations, and its contract was terminated in 2007.[24] NASA later re-awarded Kistler's contract to Orbital Sciences Corporation.[24][25]
Commercial Resupply Services Phase 1[edit]
On 23 December 2008, NASA awarded a US$1.6 billion Commercial Resupply Services (CRS-1) contract to SpaceX, with contract options that could potentially increase the maximum contract value to US$3.1 billion.[26] The contract called for 12 flights, with an overall minimum of 20,000 kilograms (44,000 lb) of cargo to be carried to the ISS.[26]
On 23 February 2009, SpaceX announced that its chosen phenolic-impregnated carbon ablator heat shield material, PICA-X, had passed heat stress tests in preparation for Dragon's maiden launch.[27][28] The primary proximity-operations sensor for the Dragon spacecraft, the DragonEye, was tested in early 2009 during the STS-127 mission, when it was mounted near the docking port of the Space Shuttle Endeavour and used while the Shuttle approached the International Space Station. The DragonEye's lidar and thermography (thermal imaging) abilities were both tested successfully.[29][30] The COTS UHF Communication Unit (CUCU) and Crew Command Panel (CCP) were delivered to the ISS during the late 2009 STS-129 mission.[31] The CUCU allows the ISS to communicate with Dragon and the CCP allows ISS crew members to issue basic commands to Dragon.[31] In summer 2009, SpaceX hired former NASA astronaut Ken Bowersox as vice president of their new Astronaut Safety and Mission Assurance Department, in preparation for crews using the spacecraft.[32]
As a condition of the NASA CRS contract, SpaceX analyzed the orbital radiation environment on all Dragon systems, and how the spacecraft would respond to spurious radiation events. That analysis and the Dragon design – which uses an overall Fault tolerancetriple redundantcomputer architecture, rather than individual radiation hardening of each computer processor – was reviewed by independent experts before being approved by NASA for the cargo flights.[33]
During March 2015, it was announced that SpaceX had been awarded an additional three missions under Commercial Resupply Services Phase 1.[34] These additional missions are SpaceX CRS-13, SpaceX CRS-14 and SpaceX CRS-15 and would cover the cargo needs of 2017. On 24 February 2016, SpaceNews disclosed that SpaceX had been awarded a further five missions under Commercial Resupply Services Phase 1.[35] This additional tranche of missions had SpaceX CRS-16 and SpaceX CRS-17 manifested for FY2017 while SpaceX CRS-18, SpaceX CRS-19 and SpaceX CRS-20 and were notionally manifested for FY2018.
Commercial Resupply Services Phase 2[edit]
The Commercial Resupply Services-2 (CRS-2) contract definition and solicitation period commenced in 2014. In January 2016, NASA awarded contracts to SpaceX, Orbital ATK, and Sierra Nevada Corporation for a minimum of six launches each, with missions planned until at least 2024. The maximum potential value of all the contracts was announced as US$14 billion, but the minimum requirements would be considerably less.[36] No further financial information was disclosed.
CRS-2 launches began in late 2019.
Demonstration flights[edit]



The first flight of the Falcon 9, a private flight, occurred in June 2010 and launched a stripped-down version of the Dragon capsule. This Dragon Spacecraft Qualification Unit had initially been used as a ground test bed to validate several of the capsule's systems. During the flight, the unit's primary mission was to relay aerodynamic data captured during the ascent.[37][38] It was not designed to survive re-entry, and did not.
NASA contracted for three test flights from SpaceX, but later reduced that number to two. The first Dragon spacecraft launched on its first mission – contracted to NASA as COTS Demo Flight 1 – on 8 December 2010, and was successfully recovered following re-entry to Earth's atmosphere. The mission also marked the second flight of the Falcon 9 launch vehicle.[39] The DragonEye sensor flew again on STS-133 in February 2011 for further on-orbit testing.[40] In November 2010, the Federal Aviation Administration (FAA) had issued a re-entry license for the Dragon capsule, the first such license ever awarded to a commercial vehicle.[41]
The second Dragon flight, also contracted to NASA as a demonstration mission, launched successfully on 22 May 2012, after NASA had approved SpaceX's proposal to combine the COTS 2 and 3 mission objectives into a single Falcon 9/Dragon flight, renamed COTS 2+.[4][42] Dragon conducted orbital tests of its navigation systems and abort procedures, before being grappled by the ISS' Canadarm2 and successfully berthing with the station on 25 May 2012 to offload its cargo.[8][43][44][45][46] Dragon returned to Earth on 31 May 2012, landing as scheduled in the Pacific Ocean, and was again successfully recovered.[47][48]
On 23 August 2012, NASA Administrator Charles Bolden announced that SpaceX had completed all required milestones under the COTS contract, and was cleared to begin operational resupply missions to the ISS.[49]
Returning research materials from orbit[edit]
Dragon spacecrafts can return to Earth 3,500 kilograms (7,700 lb), which can be all unpressurized disposal mass or up to 3,000 kilograms (6,600 lb) of return pressurized cargo from the ISS,[2] and is the only current spacecraft capable of returning to Earth with a significant amount of cargo. Other than the Russian Soyuz crew capsule, Dragon is the only currently operating spacecraft designed to survive re-entry. Because Dragon allows for the return of critical materials to researchers in as little as 48 hours from splashdown, it opens the possibility of new experiments on ISS that can produce materials for later analysis on ground using more sophisticated instrumentation. For example, CRS-12 returned mice that have spent time in orbit which will help give insight into how microgravity impacts blood vessels in both the brain and eyes, and in determining how arthritis develops.[50]
Operational flights[edit]
Dragon was launched on its first operational CRS flight on 8 October 2012,[11] and completed the mission successfully on 28 October 2012.[51]NASA initially contracted SpaceX for 12 operational missions, and later extended the CRS contract with 8 more flights, bringing the total to 20 launches until 2019. In 2016, a new batch of 6 missions under the CRS-2 contract was assigned to SpaceX; those missions are scheduled to be launched between 2020 and 2024.
Reuse of previously-flown capsules[edit]
SpaceX CRS-11, SpaceX's eleventh CRS mission, was successfully launched on 3 June 2017 from Kennedy Space CenterLC-39A, being the 100th mission to be launched from that pad. This mission was the first to re-fly a recovered Dragon capsule that previously flew on CRS-4 mission. This mission delivered 2,708 kilograms[52] of cargo to the International Space Station, including Neutron Star Interior Composition Explorer (NICER).[53] The first stage of the Falcon 9 launch vehicle landed successfully at Landing Zone 1. This mission launched for the first time a refurbished Dragon capsule,[54] serial number C106, which had flown in September 2014 on the CRS-4 mission,[55] and was the first time since 2011 a reused spacecraft arrived at the ISS.[56]Gemini SC-2 capsule is the only other reused capsule, but it was only reflown suborbitally in 1966.
SpaceX CRS-12, SpaceX's twelfth CRS mission, was successfully launched on the first "Block 4" version of the Falcon 9 on 14 August 2017 from Kennedy Space CenterLC-39A at the first attempt. This mission delivered 2,349 kilograms (5,179 lb) of pressurized mass and 961 kilograms (2,119 lb) unpressurized. The external payload manifested for this flight was the CREAM cosmic-ray detector. Last flight of a newly built Dragon capsule; further missions will use refurbished spacecraft.[57]
SpaceX CRS-13, SpaceX's thirteenth CRS mission, was the second use of a previously-flown Dragon capsule, but the first time in concordance with a reused first-stage booster. It was successfully launched on 15 December 2017 from Cape Canaveral Air Force StationSpace Launch Complex 40 at the first attempt. This was the first launch from SLC-40 since the Amos-6 pad anomaly. The booster was the previously-flown core from the CRS-11 mission. This mission delivered 1,560 kilograms (3,440 lb) of pressurized mass and 645 kilograms (1,422 lb) unpressurized. It returned from orbit and splashdown on 13 January 2018, making it the first space capsule to be reflown to orbit more than once.[58]
SpaceX CRS-14, SpaceX's fourteenth CRS mission, was the third reuse of a previously-flown Dragon capsule. It was successfully launched on 2 April 2018 from Cape Canaveral Air Force StationSLC-40. It successfully docked with the ISS on 4 April 2018 and remained docked for a month before returning cargo and science experiments back to Earth.
SpaceX CRS-15, SpaceX CRS-16, SpaceX CRS-17, SpaceX CRS-18, SpaceX CRS-19, and SpaceX CRS-20 were all flown with previously flown capsules.
Crewed development program[edit]

In 2006, Elon Musk stated that SpaceX had built "a prototype flight crew capsule, including a thoroughly tested 30-man-day life-support system".[20] A video simulation of the launch escape system's operation was released in January 2011.[59] Musk stated in 2010 that the developmental cost of a crewed Dragon and Falcon 9 would be between US$800 million and US$1 billion.[60] In 2009 and 2010, Musk suggested on several occasions that plans for a crewed variant of the Dragon were proceeding and had a two-to-three-year timeline to completion.[61][62] SpaceX submitted a bid for the third phase of CCDev, CCiCap.[63][64]
Development funding[edit]
In 2014, SpaceX released the total combined development costs for both the Falcon 9 launch vehicle and the Dragon capsule. NASA provided US$396 million while SpaceX provided over US$450 million to fund both development efforts.[65]
Production[edit]

In December 2010, the SpaceX production line was reported to be manufacturing one new Dragon spacecraft and Falcon 9 rocket every three months. Elon Musk stated in a 2010 interview that he planned to increase production turnover to one Dragon every six weeks by 2012.[66]Composite materials are extensively used in the spacecraft's manufacture to reduce weight and improve structural strength.[67]
By September 2013, SpaceX total manufacturing space had increased to nearly 1,000,000 square feet (93,000 m2) and the factory had six Dragons in various stages of production. SpaceX published a photograph showing the six, including the next four NASA Commercial Resupply Services (CRS-1) mission Dragons (CRS-3, CRS-4, CRS-5, CRS-6) plus the drop-test Dragon, and the pad-abort Dragon weldment for commercial crew program.[68]
Design[edit]

The Dragon spacecraft consists of a nose-cone cap, a conventional blunt-cone ballistic capsule, and an unpressurized cargo-carrier trunk equipped with two solar arrays.[69] The capsule uses a PICA-X heat shield, based on a proprietary variant of NASA's Phenolic impregnated carbon ablator (PICA) material, designed to protect the capsule during Earthatmospheric entry, even at high return velocities from Lunar and Martian missions.[70][71][72] The Dragon capsule is re-usable, and can fly multiple missions.[69] The trunk is not recoverable; it separates from the capsule before re-entry and burns up in Earth's atmosphere.[73] The trunk section, which carries the spacecraft's solar panels and allows the transport of unpressurized cargo to the ISS, was first used for cargo on the SpaceX CRS-2 mission.
The spacecraft is launched atop a Falcon 9 booster.[74] The Dragon capsule is equipped with 18 Draco thrusters.[71] During its initial cargo and crew flights, the Dragon capsule will land in the Pacific Ocean and be returned to the shore by ship.[75]
For the ISS Dragon cargo flights, the ISS's Canadarm2 grapples its Flight-Releasable Grapple Fixture and berths Dragon to the station's US Orbital Segment using a Common Berthing Mechanism (CBM).[76] The CRS Dragon does not have an independent means of maintaining a breathable atmosphere for astronauts and instead circulates in fresh air from the ISS.[77] For typical missions, Dragon is planned to remain berthed to the ISS for about 30 days.[78]
The Dragon capsule can transport 3,310 kilograms (7,300 lb) of cargo, which can be all pressurized, all unpressurized, or a combination thereof. It can return to Earth 3,310 kilograms (7,300 lb), which can be all unpressurized disposal mass, or up to 3,310 kilograms (7,300 lb) of return pressurized cargo, driven by parachute limitations. There is a volume constraint of 14 cubic metres (490 cu ft) trunk unpressurized cargo and 11.2 cubic metres (400 cu ft) of pressurized cargo (up or down).[79] The trunk was first used operationally on the Dragon's CRS-2 mission in March 2013.[80] Its solar arrays produce a peak power of 4 kW.[5]
The design was modified beginning with the fifth Dragon flight on the SpaceX CRS-3 mission to the ISS in March 2014. While the outer mold line of the Dragon was unchanged, the avionics and cargo racks were redesigned to supply substantially more electrical power to powered cargo devices, including the GLACIER freezer module and MERLIN freezer module freezer modules for transporting critical science payloads.[81]
Variants and derivatives[edit]
DragonLab[edit]
When used for non-NASA, non-ISS commercial flights, the uncrewed version of the Dragon spacecraft is called DragonLab.[69] It is reusable and free-flying and can carry pressurized and unpressurized payloads. Its subsystems include propulsion, power, thermal and environmental control (ECLSS), avionics, communications, thermal protection, flight software, guidance and navigation systems, and entry, descent, landing, and recovery gear.[3] It has a total combined upmass of 6,000 kilograms (13,000 lb) upon launch, and a maximum downmass of 3,000 kilograms (6,600 lb) when returning to Earth.[3] In November 2014, there were two DragonLab missions listed on the SpaceX launch manifest: one in 2016 and another in 2018.[82] However, these missions were removed from the manifest in early 2017, with no official SpaceX statement.[83] The American Biosatellites once performed similar uncrewed payload-delivery functions, and the Russian Bion satellites still continue to do so.
Dragon 2: Crew and Cargo[edit]
A successor of Dragon called SpaceX Dragon 2 has been developed by SpaceX, designed to carry passengers and crew. It has been designed to be able to carry up to seven astronauts, or some mix of crew and cargo, to and from low Earth orbit.[84] The Dragon 2 heat shield is designed to withstand Earth re-entry velocities from Lunar and Martian spaceflights.[70] SpaceX undertook several U.S. Government contracts to develop the Dragon 2 crewed variant, including a Commercial Crew Development 2 (CCDev 2) - funded Space Act Agreement in April 2011, and a Commercial Crew integrated Capability (CCiCap) - funded space act agreement in August 2014.[85] The phase 2 of the CRS contract will be flown using the Dragon 2 Cargo variant lacking cockpit controls, seats and life support systems.[86]
Red Dragon[edit]
Red Dragon was a cancelled version of the Dragon spacecraft that had been previously proposed to fly farther than Earth orbit and transit to Mars via interplanetary space. In addition to SpaceX's own privately funded plans for an eventual Mars mission, NASAAmes Research Center had developed a concept called Red Dragon: a low-cost Mars mission that would use Falcon Heavy as the launch vehicle and trans-Martian injection vehicle, and the SpaceX Dragon 2-based capsule to enter the atmosphere of Mars. The concept was originally envisioned for launch in 2018 as a NASA Discovery mission, then alternatively for 2022, but was never formally submitted for funding within NASA.[87] The mission would have been designed to return samples from Mars to Earth at a fraction of the cost of NASA's own sample-return mission, which was projected in 2015 to cost US$6 billion.[87]
On 27 April 2016, SpaceX announced its plan to go ahead and launch a modified Dragon lander to Mars in 2018.[88][89] However, Musk canceled the Red Dragon program in July 2017 to focus on developing the Starship system instead.[90][91] The modified Red Dragon capsule would have performed all entry, descent and landing (EDL) functions needed to deliver payloads of 1,000 kilograms (2,200 lb) or more to the Martian surface without using a parachute. Preliminary analysis showed that the capsule's atmospheric drag would slow it enough for the final stage of its descent to be within the abilities of its SuperDraco retro-propulsion thrusters.[92][93]
Dragon XL[edit]
On 27 March 2020, SpaceX revealed the Dragon XL resupply spacecraft to carry pressurized and unpressurized cargo, experiments and other supplies to NASA's planned Gateway under a Gateway Logistics Services (GLS) contract.[94] The equipment delivered by Dragon XL missions could include sample collection materials, spacesuits and other items astronauts may need on the Gateway and on the surface of the Moon, according to NASA. It will launch on SpaceX Falcon Heavy rockets from LC-39A at the Kennedy Space Center in Florida. The Dragon XL will stay at the Gateway for 6 to 12 months at a time, when research payloads inside and outside the cargo vessel could be operated remotely, even when crews are not present.[95] Its payload capacity is expected to be more than 5,000 kilograms (11,000 lb) to lunar orbit.[96]
List of missions[edit]
List includes only completed or currently manifested missions. Launch dates are listed in UTC.
| Mission | Capsule No.[97] | Launch date (UTC) | Remarks | Time at ISS (dd:hh) | Outcome |
|---|---|---|---|---|---|
| SpX-C1 | C101[98] | 8 December 2010 [99] | First Dragon mission, second Falcon 9 launch. Mission tested the orbital maneuvering and reentry of the Dragon capsule. After recovery, the capsule was put on display at SpaceX's headquarters.[98] | N/A | Success |
| SpX-C2+ | C102 | 22 May 2012 [4] | First Dragon mission with complete spacecraft, first rendezvous mission, first berthing with ISS. After recovery, the capsule was put on display at Kennedy Space Center Visitor Complex.[100] | 05d 16h | Success [47] |
| CRS-1 | C103 | 8 October 2012 [12] | First Commercial Resupply Services (CRS) mission for NASA, first non-demo mission. Falcon 9 rocket suffered a partial engine failure during launch but was able to deliver Dragon into orbit.[11] However, a secondary payload did not reach its correct orbit.[101][13][102] | 17d 22h | Success; launch anomaly [51] |
| CRS-2 | C104 | 1 March 2013 [103][104] | First launch of Dragon using trunk section to carry cargo.[80] Launch was successful, but anomalies occurred with the spacecraft's thrusters shortly after liftoff. Thruster function was later restored and orbit corrections were made,[103] but the spacecraft's rendezvous with the ISS was delayed from its planned date of 2 March until 3 March 2013, when it was successfully berthed with the Harmony module.[105][106] Dragon splashed down safely in the Pacific Ocean on 26 March 2013.[107] | 22d 18h | Success; spacecraft anomaly[103] |
| CRS-3 | C105 | 18 April 2014 [108][109] | First launch of the redesigned Dragon: same outer mold line with the avionics and cargo racks redesigned to supply substantially more electric power to powered cargo devices, including additional cargo freezers (GLACIER freezer module (GLACIER), Minus Eighty Degree Laboratory Freezer for ISS (MERLIN)) for transporting critical science payloads.[81] Launch rescheduled for 18 April 2014 due to a helium leak. | 27d 21h | Success [110] |
| CRS-4 | C106[111] | 21 September 2014 [112] | First launch of a Dragon with living payload, in the form of 20 mice which are part of a NASA experiment to study the physiological effects of long-duration spaceflight.[113] | 31d 22h | Success [114] |
| CRS-5 | C107 | 10 January 2015 [112] | Cargo manifest change due to Cygnus CRS Orb-3 launch failure.[115] Carried the Cloud Aerosol Transport System experiment. | 29d 03h | Success |
| CRS-6 | C108[111] | 14 April 2015 | The robotic SpaceX Dragon capsule splashed down in the Pacific Ocean on 21 May 2015. | 33d 20h | Success |
| CRS-7 | C109 | 28 June 2015 [116] | This mission was supposed to deliver the first of two International Docking Adapters (IDA) to modify Russian APAS-95 docking ports to the newer international standard. The payload was lost due to an in-flight explosion of the carrier rocket. The Dragon capsule survived the blast; it could have deployed its parachutes and performed a splashdown in the ocean, but its software did not take this situation into account.[117] | N/A | Failure |
| CRS-8 | C110 | 8 April 2016 [118] | Delivered the Bigelow AerospaceBigelow Expandable Activity Module (BEAM) module in the unpressurized cargo trunk.[119] First stage landed for the first time successfully on sea barge. A month later, the Dragon capsule was recovered, carrying a downmass containing astronaut's Scott Kelly biological samples from his year-long mission on board of ISS.[120] | 30d 21h | Success [121] |
| CRS-9 | C111 | 18 July 2016 [122] | Delivered docking adapter International Docking Adapter (IDA-2) to modify the ISS docking port Pressurized Mating Adapter (PMA-2) for Commercial Crew spacecraft. Longest time a Dragon Capsule was in space. | 36d 06h | Success |
| CRS-10 | C112 | 19 February 2017 [123] | First launch from Kennedy Space CenterLC-39A since STS-135 in mid-2011. Berthing to the ISS was delayed by a day due to software incompatibilities.[124] | 23d 08h | Success [125] |
| CRS-11 | C106.2♺[111] | 3 June 2017 | The first mission to re-fly a recovered Dragon capsule (previously flown on SpaceX CRS-4). | 27d 01h | Success [126] |
| CRS-12 | C113 | 14 August 2017 | Last mission to use a new Dragon 1 spacecraft. | 30 days and 21 hours | Success |
| CRS-13 | C108.2 ♺[111] | 15 December 2017 [127] | Second reuse of Dragon capsule. First NASA mission to fly aboard reused Falcon 9.[127] First reuse of this specific Dragon spacecraft. | 25 days and 21 hours | Success |
| CRS-14 | C110.2 ♺ | 2 April 2018 | Third reuse of a Dragon capsule, only necessitated replacing its heatshield, trunk, and parachutes.[128] Returned over 4000 pounds of cargo.[129] First reuse of this specific Dragon spacecraft. | 23 days and 1 hour | Success |
| CRS-15 | C111.2 ♺[130] | 29 June 2018 [131] | Fourth reuse. First reuse of this specific Dragon spacecraft. | 32 days and 2 hours | Success [132] |
| CRS-16 | C112.2 ♺[133] | 5 December 2018 [134] | Fifth reuse. First reuse of this specific Dragon spacecraft. The first-stage booster landing failed due to a grid fin hydraulic pump stall on reentry.[134] | 36 days and 7 hours | Success [135] |
| CRS-17 | C113.2 ♺[136] | 4 May 2019 |
What’s New in the Cubics Plane v1.0 serial key or number?
Screen Shot
System Requirements for Cubics Plane v1.0 serial key or number
- First, download the Cubics Plane v1.0 serial key or number
-
You can download its setup from given links:





















